Template:Example: Weibull Disribution Conditional Reliability RRX Example: Difference between revisions
Jump to navigation
Jump to search
No edit summary |
No edit summary |
||
| Line 7: | Line 7: | ||
The conditional reliability is given by: | The conditional reliability is given by: | ||
::<math>R(t|T)=\frac{R(T+t)}{R(T)}</math> | |||
or: | or: | ||
::<math>\hat{R}(10hr|30hr)=\frac{\hat{R}(10+30)}{\hat{R}(30)}=\frac{\hat{R}(40)}{\hat{R}(30)}</math> | |||
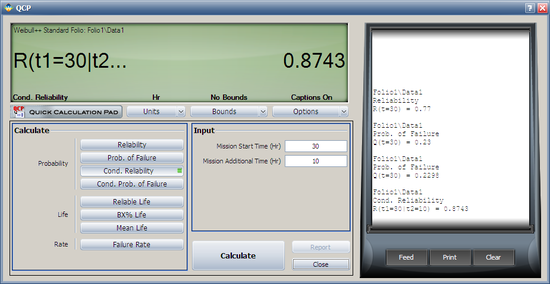
Again, the '''Quick Calculation Pad''' can provide this result directly and more accurately than the plot. | Again, the '''Quick Calculation Pad''' can provide this result directly and more accurately than the plot. | ||
[[Image: Conditional R.png | [[Image: Conditional R.png|center|550px]] | ||
Revision as of 05:29, 6 August 2012
Weibull Disribution Conditional Reliability RRX Example
What is the reliability for a new mission of t = 10 hours duration, starting the new mission at the age of T = 30 hours, for the same data as Example 8?
Solution
The conditional reliability is given by:
- [math]\displaystyle{ R(t|T)=\frac{R(T+t)}{R(T)} }[/math]
or:
- [math]\displaystyle{ \hat{R}(10hr|30hr)=\frac{\hat{R}(10+30)}{\hat{R}(30)}=\frac{\hat{R}(40)}{\hat{R}(30)} }[/math]
Again, the Quick Calculation Pad can provide this result directly and more accurately than the plot.