|
Cumulative Damage Model for Step Stress Profiles
|
This example compares the results of a cumulative damage model for a step stress test.
Reference Case
The data set is from Table 2.1 on page 496 in the book Accelerated Testing: Statistical Models, Test Plans, and Data Analysis by Dr. Nelson, John Wiley & Sons, 1990.
Data
A step-stress test is conducted for one type of cable insulation to estimate the insulation life at a constant design stress of 400 volts/mil. The test cables are of different thickness, in unit of mils (0.001 inch). The stress is the applied step voltage divided by the thickness.
A total of 6 step-stress profiles are used. These stress profiles are calculated based on the applied voltage (Kilovolts) and the insulation thickness given on page 495 and Table 2.1. For all the stress profiles, the holding time for the first 4 steps is 10 mins. From step 5 onwards, a different a holding time is applied at each step for each of the stress profiles. These profiles are given in the following tables.
Profile 1 (named "G1"): The holding time after step 4 is 15 mins and the thickness is 27 mils.
| Segment Start Time
|
Segment End Time
|
Stress (Kvolts/mils)
|
| 0 |
10 |
0.185185185
|
| 10 |
20 |
0.37037037
|
| 20 |
30 |
0.555555556
|
| 30 |
40 |
0.740740741
|
| 40 |
55 |
0.962962963
|
| 55 |
70 |
1.055555556
|
| 70 |
85 |
1.148148148
|
| 85 |
100 |
1.233333333
|
| 100 |
115 |
1.333333333
|
| 115 |
130 |
1.425925926
|
| 130 |
145 |
1.518518519
|
Profile 2 (named "G2"): The holding time after step 4 is 60 mins and the thickness is 29.5 mils.
| Segment Start Time
|
Segment End Time
|
Stress (Kvolts/mils)
|
| 0 |
10 |
0.169491525
|
| 10 |
20 |
0.338983051
|
| 20 |
30 |
0.508474576
|
| 30 |
40 |
0.677966102
|
| 40 |
100 |
0.881355932
|
| 100 |
160 |
0.966101695
|
| 160 |
220 |
1.050847458
|
| 220 |
280 |
1.128813559
|
| 280 |
340 |
1.220338983
|
| 340 |
400 |
1.305084746
|
| 400 |
460 |
1.389830508
|
Profile 3 (named "G3"): The holding time after step 4 is 60 mins and the thickness is 28 mils.
| Segment Start Time
|
Segment End Time
|
Stress (Kvolts/mils)
|
| 0 |
10 |
0.178571429
|
| 10 |
20 |
0.357142857
|
| 20 |
30 |
0.535714286
|
| 30 |
40 |
0.714285714
|
| 40 |
100 |
0.928571429
|
| 100 |
160 |
1.017857143
|
| 160 |
220 |
1.107142857
|
| 220 |
280 |
1.189285714
|
| 280 |
340 |
1.285714286
|
| 340 |
400 |
1.375
|
| 400 |
460 |
1.464285714
|
Profile 4 (named "G4"): The holding time after step 4 is 240 mins and the thickness is 29 mils.
| Segment Start Time
|
Segment End Time
|
Stress (Kvolts/mils)
|
| 0 |
10 |
0.172413793
|
| 10 |
20 |
0.344827586
|
| 20 |
30 |
0.517241379
|
| 30 |
40 |
0.689655172
|
| 40 |
280 |
0.896551724
|
| 280 |
520 |
0.982758621
|
| 520 |
760 |
1.068965517
|
| 760 |
1000 |
1.148275862
|
| 1000 |
1240 |
1.24137931
|
| 1240 |
1480 |
1.327586207
|
| 1480 |
1720 |
1.413793103
|
Profile 5 (named "G5"): The holding time after step 4 is 240 mins and the thickness is 30 mils.
| Segment Start Time
|
Segment End Time
|
Stress (Kvolts/mils)
|
| 0 |
10 |
0.166666667
|
| 10 |
20 |
0.333333333
|
| 20 |
30 |
0.5
|
| 30 |
40 |
0.666666667
|
| 40 |
280 |
0.866666667
|
| 280 |
520 |
0.95
|
| 520 |
760 |
1.033333333
|
| 760 |
1000 |
1.11
|
| 1000 |
1240 |
1.2
|
| 1240 |
1480 |
1.283333333
|
| 1480 |
1720 |
1.366666667
|
Profile 6 (named "G6"): The holding time after step 4 is 960 mins and the thickness is 30 mils.
| Segment Start Time
|
Segment End Time
|
Stress (Kvolts/mils)
|
| 0 |
10 |
0.166666667
|
| 10 |
20 |
0.333333333
|
| 20 |
30 |
0.5
|
| 30 |
40 |
0.666666667
|
| 40 |
1000 |
0.866666667
|
| 1000 |
1960 |
0.95
|
| 1960 |
2920 |
1.033333333
|
| 2920 |
3880 |
1.11
|
| 3880 |
4840 |
1.2
|
| 4840 |
5800 |
1.283333333
|
| 5800 |
6760 |
1.366666667
|
The following table shows the test results.
| Status F/S
|
Time to F/S
|
Test Profile
|
Subset ID
|
| F |
102 |
G1 |
1
|
| F |
113 |
G1 |
2
|
| F |
113 |
G1 |
3
|
| S |
370 |
G2 |
4
|
| F |
345 |
G2 |
5
|
| S |
345 |
G3 |
6
|
| F |
1249 |
G4 |
7
|
| F |
1333 |
G4 |
8
|
| S |
1333 |
G4 |
9
|
| F |
1096.6 |
G4 |
10
|
| F |
1250.8 |
G5 |
11
|
| F |
1097.9 |
G4 |
12
|
| F |
2460.9 |
G6 |
13
|
| S |
2460.9 |
G6 |
14
|
| F |
2700.4 |
G6 |
15
|
| F |
2923.9 |
G6 |
16
|
| F |
1160 |
G6 |
17
|
| F |
1962.9 |
G6 |
18
|
| S |
363.9 |
G6 |
19
|
| F |
898.4 |
G6 |
20
|
| F |
4142.1 |
G6 |
21
|
Result
The power law life stress relationship and the Weibull distribution is used to analyze the data. At a constant stress V, the [math]\displaystyle{ \eta\,\! }[/math] is:
- [math]\displaystyle{ \eta(V) = \left(\frac{V_{0}}{V} \right)^p\,\! }[/math]
where [math]\displaystyle{ V_{0}\,\! }[/math] and [math]\displaystyle{ p\,\! }[/math] are the model parameters used in the book.
The above equation can be rewritten as:
- [math]\displaystyle{ \eta(V) = e^{\alpha_{0}+\alpha_{1}ln(V)}\,\! }[/math]
where [math]\displaystyle{ \alpha_{0} = pln(V_{0})\,\! }[/math] and [math]\displaystyle{ \alpha_{1} = -p\,\! }[/math]
The reliability function at time t and stress V is:
- [math]\displaystyle{ R(t,V) = e^{-\left(\frac{t}{\eta(V)} \right)^\beta}\,\! }[/math]
When stress is varying with time, the reliability at time t is given as:
- [math]\displaystyle{ R(t,V) = e^{-\left(\int_{0}^{t}\frac{1}{\eta(x)} dx\right)^{\beta}}\,\! }[/math]
In the book, the following results are provided:
- ML solution for the parameters are [math]\displaystyle{ \beta\,\! }[/math] = 0.75597, [math]\displaystyle{ V_{0}\,\! }[/math] = 1616.4 (1.6164 Kvolts), and [math]\displaystyle{ p\,\! }[/math] = 19.937.
- The maximum log likelihood is -103.53.
- The 1% percentile point (B1 life) at 0.4 Kvolts/mil is 2.81 x 109.
- The normal distribution approximation two-sided 95% confidence intervals are [math]\displaystyle{ \beta\,\! }[/math] = [0.18, 1.33], [math]\displaystyle{ V_{0}\,\! }[/math] = [1291, 1941.8], [math]\displaystyle{ p\,\! }[/math] = [6.2, 33.7], and the B1 life is [2.65 x 104, 2.98 x 1014].
Results in ALTA
First, we create each stress profile in ALTA. For example, the following picture shows the data for Profile G1.
The following picture shows the plot for this stress profile.
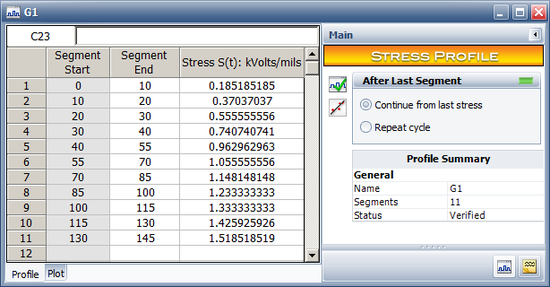
The next step is to enter the failure data into an ALTA standard folio and use the stress profiles to define the stress values, as shown next.
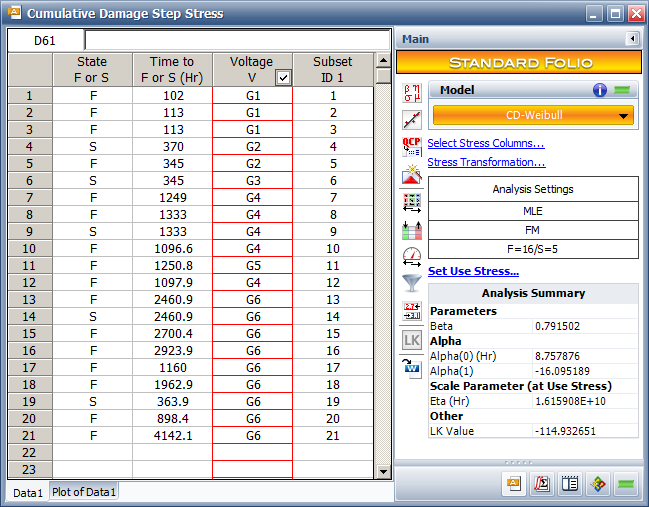
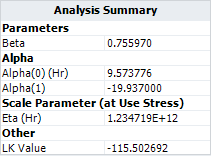
The log transformation is used for the stress. To compare the parameter values used in the book with the results obtained by ALTA, we need do convert the values in the book in terms of the parameters used in ALTA. Therefore the parameters in the book are: [math]\displaystyle{ \beta\,\! }[/math] = 0.75597, [math]\displaystyle{ \alpha_{0}\,\! }[/math] = 9.573776, [math]\displaystyle{ \alpha_{1}\,\! }[/math] = -19.937. Comparing these values to the value shown in the picture above, we see that the results obtained in ALTA are close to the results shown in the book.
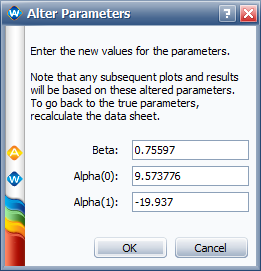
The picture above also shows that the maximum log likelihood (LK Value) is -114.932651. This value is smaller than the value given in the book. To validate what the LK value would be if the results in the book were used in ALTA, we use the Alter Parameters tool, as shown next.
The resulting LK Value is -115.502692, as shown next.
Altered Parameters
This value is different from the value of -13.53 given in the book. Later, we will validate the calculations for this result.
Using the parameters values originally calculated in ALTA:
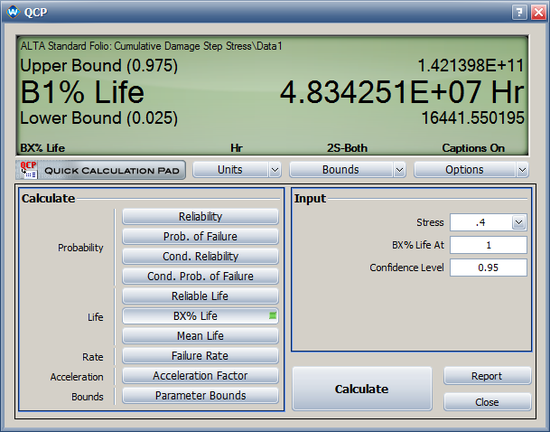
- The 1% percentile point (B1 life) at 0.4 Kvolts/mil is 4.8342 X 107 hours, as shown next.
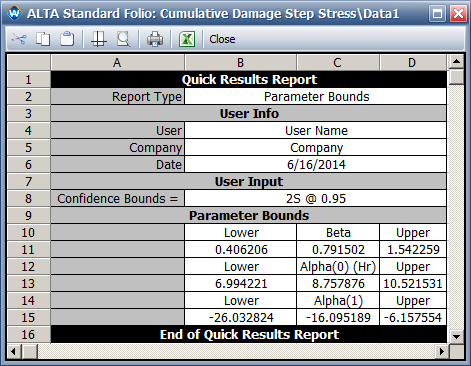
- The 2-sided 95% confidence intervals for model parameters are shown next.
Validate the Likelihood Calculation in ALTA
