Stress-Strength Analysis in Design for Reliability: Difference between revisions
No edit summary |
Lisa Hacker (talk | contribs) No edit summary |
||
| (4 intermediate revisions by 3 users not shown) | |||
| Line 1: | Line 1: | ||
<noinclude>{{Banner Weibull Examples}} | <noinclude>{{Banner Weibull Examples}} | ||
''This example appears in the [ | ''This example appears in the [https://help.reliasoft.com/reference/life_data_analysis Life data analysis reference]''. | ||
</noinclude> | </noinclude> | ||
<!-- THIS PAGE IS LINKED TO THE WEIBULL++/ALTA8 HELP FILE --> | |||
Assume that the stress distribution for a component is known to be a Weibull distribution with beta = 3 and eta = 2000. For the current design, the strength distribution is also a Weibull distribution with beta =1.5 and eta=4000. Evaluate the current reliability of the component. If the reliability does not meet the target reliability of 90%, determine what parameters would be required for the strength distribution in order to meet the specified target. | Assume that the stress distribution for a component is known to be a Weibull distribution with beta = 3 and eta = 2000. For the current design, the strength distribution is also a Weibull distribution with beta =1.5 and eta=4000. Evaluate the current reliability of the component. If the reliability does not meet the target reliability of 90%, determine what parameters would be required for the strength distribution in order to meet the specified target. | ||
'''Solution''' | '''Solution''' | ||
| Line 10: | Line 10: | ||
The following picture shows the stress-strength tool and the calculated reliability of the current design. | The following picture shows the stress-strength tool and the calculated reliability of the current design. | ||
[[Image: Stress-strength example 2 current reliability.png|center|700px]] | |||
[[Image: Stress-strength example 2 current reliability.png | |||
The result shows that the current reliability is about 74.0543%, which is below the target value of 90%. We need to use the '''Target Reliability Parameter Estimator''' to determine the parameters for the strength distribution that, when compared against the stress distribution, would result in the target reliability. | The result shows that the current reliability is about 74.0543%, which is below the target value of 90%. We need to use the '''Target Reliability Parameter Estimator''' to determine the parameters for the strength distribution that, when compared against the stress distribution, would result in the target reliability. | ||
| Line 18: | Line 16: | ||
The following picture shows the Target Reliability Parameter Estimator window. In the '''Strength Parameters''' area, select '''eta'''. Set the Target Reliability to '''90%''' and click '''Calculate'''. The calculated eta is 8192.2385 hours. | The following picture shows the Target Reliability Parameter Estimator window. In the '''Strength Parameters''' area, select '''eta'''. Set the Target Reliability to '''90%''' and click '''Calculate'''. The calculated eta is 8192.2385 hours. | ||
[[Image:Stress-strength example 2 Result.png|center|650px]] | |||
[[Image:Stress-strength example 2 Result.png | |||
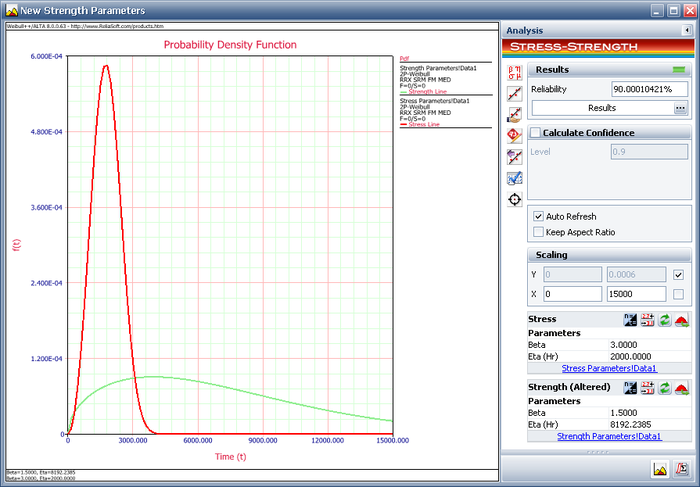
Click '''Update''' to perform the stress-strength analysis again using the altered parameters for the strength distribution. The following plot shows that the calculated reliability is 90%. Therefore, in order to meet the reliability requirement, the component must be redesigned such that the eta parameter of the strength distribution is at least 8192.2385 hours. | Click '''Update''' to perform the stress-strength analysis again using the altered parameters for the strength distribution. The following plot shows that the calculated reliability is 90%. Therefore, in order to meet the reliability requirement, the component must be redesigned such that the eta parameter of the strength distribution is at least 8192.2385 hours. | ||
[[Image:Stress-strength example 2 Confirmed Result.png|center|700px]] | |||
[[Image:Stress-strength example 2 Confirmed Result.png | |||
Latest revision as of 18:54, 18 September 2023
 |
New format available! This reference is now available in a new format that offers faster page load, improved display for calculations and images and more targeted search.
As of January 2024, this Reliawiki page will not continue to be updated. Please update all links and bookmarks to the latest references at Weibull examples and Weibull reference examples.
This example appears in the Life data analysis reference.
Assume that the stress distribution for a component is known to be a Weibull distribution with beta = 3 and eta = 2000. For the current design, the strength distribution is also a Weibull distribution with beta =1.5 and eta=4000. Evaluate the current reliability of the component. If the reliability does not meet the target reliability of 90%, determine what parameters would be required for the strength distribution in order to meet the specified target.
Solution
The following picture shows the stress-strength tool and the calculated reliability of the current design.
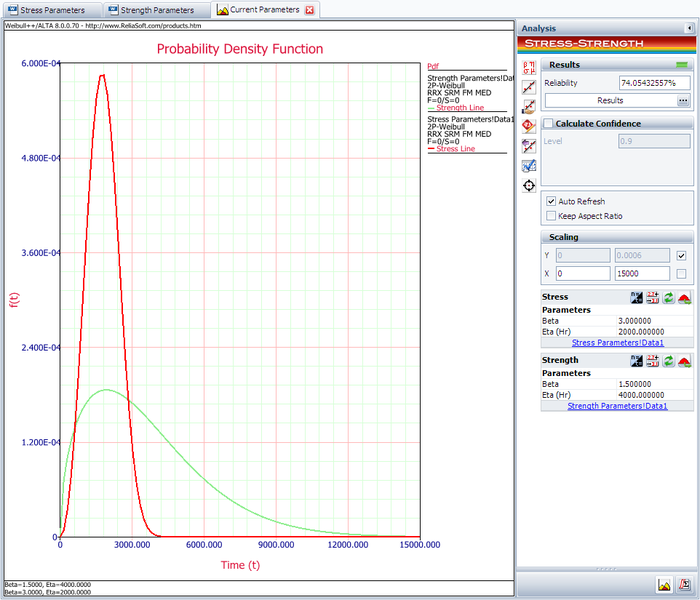
The result shows that the current reliability is about 74.0543%, which is below the target value of 90%. We need to use the Target Reliability Parameter Estimator to determine the parameters for the strength distribution that, when compared against the stress distribution, would result in the target reliability.
The following picture shows the Target Reliability Parameter Estimator window. In the Strength Parameters area, select eta. Set the Target Reliability to 90% and click Calculate. The calculated eta is 8192.2385 hours.

Click Update to perform the stress-strength analysis again using the altered parameters for the strength distribution. The following plot shows that the calculated reliability is 90%. Therefore, in order to meet the reliability requirement, the component must be redesigned such that the eta parameter of the strength distribution is at least 8192.2385 hours.